Cal's Corner
Focus on Fractions: Linking Fractions and Decimals (Using the Number Line)
This is part of a series on fractions:
- Part 1: History of Fractions and Fraction Concepts
- Part 2: Fractions Facts
- Part 3: Fraction models and visuals
- Part 4: this post – decimal fractions and the number line
Decimal fractions used in history and the use of the number line in teaching fractions.
In this post, I’ll explain how fractions came into being and how today’s number line is an invaluable tool for making the link between fractions and decimals. My first post in this series described the elusiveness of fractions to previous cultures. To overcome some of the difficulties, measurement systems were created to facilitate breaking quantities into equal parts without the need for fractions. Hence, hours/minutes, yards/feet/inches, pounds/ounces, gallons/quarts/pints, and dozens were units that helped reduce the need for fractions. Ultimately, these workarounds did not offer a complete solution and the a-over-b form (a/b) for fractions was introduced. Their use was universal — everyone needed fractions and often everyday. In English, the everyday person was called a “commoner.” Hence, these were called the common fractions. Those of us who recall attending school 60 to 70 years ago will remember that these were sometimes called vulgar fractions. That’s because, when the term was first introduced, the word vulgar had a neutral meaning which equated to common.1

History of Decimal Fractions
In 1202, the Italian mathematician Fibonacci introduced decimal numbers to Europe in his seminal text, Liber Abaci. In that book, he used fractions along with the base-ten numbers, not decimals. Decimal fractions were first introduced in Arabic texts in the mid-10th century, but there was not yet a form for writing decimals.2 It took a long time for base-ten whole numbers and then decimal fractions to become popular with common people. Perhaps not surprisingly, scientists were the first to use decimal fractions. Simon Stevin (1548–1620), the Dutch-Flemish mathematician commonly known as Stevinus, is credited with the first systematic exposition that described how all numbers known up to that point in history could be written in one form using decimals.3 The use of decimal fractions was accelerated by the printing press. The a/b form for fractions was just too complicated for setting type. Even today, modern word processing does not have a “nice” way to show common fractions.
History of the Fractions Number Line
Number lines did not appear in school books until the early 1900s.5 The importance of using a number line was emphasized again in the 1960s and since 2000. In each case, mathematicians provided the impetus for using the number line. They recognized that this tool is really the only way to show the connection between those numbers discussed in elementary grades: common fractions, decimals, integers, and whole numbers. However, the line representation for numbers can be complex for students unless care is taken when it is introduced and used.
|
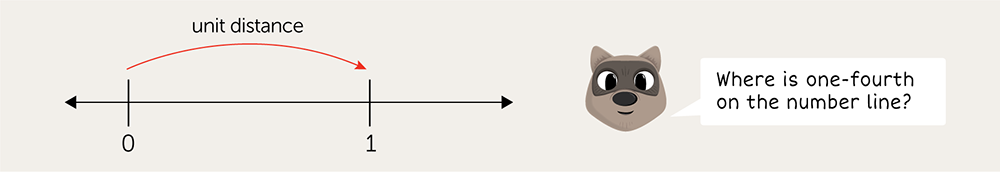
On a number line, a number is represented by a distance and direction from 0. |
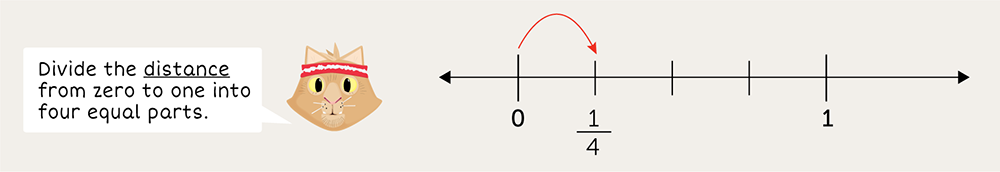
On a number line, like other models, a common fraction is identified by first dividing the distance from 0 to 1 (the unit distance) into equal parts.
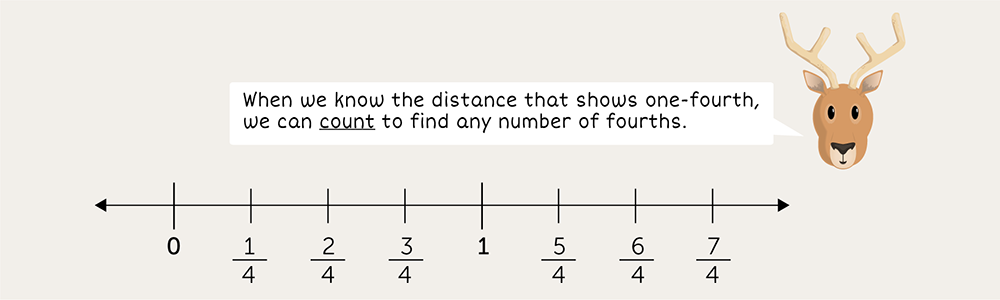
Copies of the same number line can then be used to find fractions that are equivalent to fourths. Through investigations, students find that every fourth on the line above will align with eighths, twelfths, sixteenths, twentieths, and so on. During these explorations, which involve the development of equivalent fractions,6 students will find that some, but not all, fourths will align with tenths. On the other hand, every fourth can be written as a common fraction using hundredths.

In turn, this means that every fourth has a decimal equivalent that can be expressed as an exact number of hundredths.
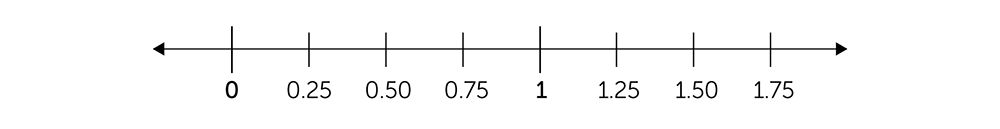
Decimal Fractions: 2 Key Properties to Understand
There are two key properties that students should understand about decimal fractions:
- First, that decimal fractions like the above,7 have an a/b common fraction form.
- Second, that decimal fractions have place value.
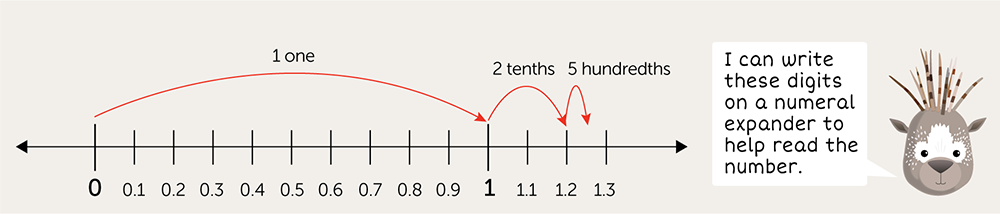
This special place-value feature can be stressed by asking students to work systematically on the number line when they investigate this property. For example, to locate the number 1.25 on the number line, students should move from place to place, working left to right, in the same way as they would to find a whole number. Therefore, the number line should be divided to highlight the place-value groupings that show ones, tenths, and hundredths. While it is not always practical to show every tenth or hundredth, the students do need to know that each distance which shows a whole number is divided into 10 equal parts called tenths. Then every tenth is divided into 10 equal parts called hundredths. Later, the same would apply to every hundredth to show thousandths.
With this information, students should work left to right using place-value names to locate 1.25. It is important to explain that the first jump is a distance of 1 whole one. This is followed by a jump of 2 tenths and then 5 hundredths.
The above discussion has outlined some key ideas to help link common and decimal fractions. Reflecting on the descriptions of the four fraction models described in Focus on Fractions: Visual Images That Help Teach Fractions, it should be clear that the number line is the one representation that clearly demonstrates the connection between the two forms for fractions and shows the important mathematical properties for these numbers.
1 Schwartzman, S. 1994. The Words of Mathematics: An Etymological Dictionary of Mathematics Terms Used in English. Washington, D.C.: The Mathematics Association of America
2 Joseph, G.G. 1991. The Crest of the Peacock: Non-European Roots of Mathematics. London: Penguin Books. p318
3 Grattan-Guinness, I. 1997. The Norton History of the Mathematical Sciences. New York: W.W. Norton & Company. p186
4 Joseph, G.G. ibid
5 Wessman-Enzinger, N. 2018. Integer Number Lines in U.S. School Mathematics – Discussion. Convergence. Mathematics Association of America
6 The development of equivalent fractions is the focus of the discussion in Blog 3.5.
7 Every decimal fraction which terminates or continues in some form of a repeating pattern can be written in an a/b common fraction form. The method to find the common fraction equivalent to a repeating decimal involves a basic understanding of algebra.




