Cal's Corner
Fraction Models : Visual Images That Help Teach Fractions
This is part of a series on fractions:
- Part 1: History of Fractions and Fraction Concepts
- Part 2: Fractions Facts
- Part 3: This Post – Fraction models and visuals
- Part 4: Decimal Fractions and the Number Line
Children benefit greatly from the use of concrete materials and visual images when learning fractions.
Over the past 50-plus years, various learning theorists have advocated the use of concrete materials and visual images to help children develop full understanding of mathematical ideas, but three stand out in particular:
- The most noted was Jean Piaget, who described stages of cognitive development involved in children’s assimilation of new ideas, using material and pictures as they work within their environment.
- Jerome Bruner wrote about the importance of children interacting with their environment to promote learning. He described 3 broad stages of progression that were observed for all children – enactive, iconic, and symbolic. While all stages involved the use of materials in some way, the iconic stage focuses on 3D objects and 2D pictures.2
- The Hungarian mathematician and learning theorist Zoltan Dienes described the use of multiple embodiments.3 He promoted the use of the full range of concrete materials and pictures that show the many features of a single mathematics concept.

Children benefit greatly from the use of concrete materials and pictures when learning fractions, which have many aspects that need to be explored and then linked together to make sense of them. The table below describes various approaches to teaching fractions with materials and pictures, and the aspects of learning they cover.
| Aspect | Questions | Situations |
| Familiarity | What everyday situations involving fractions are familiar to students? | Students share collections of objects and cut-up food (pizza). They work with pictures of sets and area. |
| Links to known content | What helps students link fractions to whole numbers? | The number line is the only viable tool that shows the link. |
| Prerequisite to future content – fractions as numbers | What knowledge or concepts do students need to know about fractions as numbers (proper fractions, improper fractions, and decimal representations)? | Length shown in a fraction wall and number line best represent improper fractions. The number line clearly shows all three forms and the links between them. |
| Prerequisite to future content – addition/ subtraction | What knowledge or concepts do students need to add/subtract fractions?
What helps students avoid misconceptions? |
Knowledge of equivalent fractions is essential to work with same denominators. This is best shown using length or number line. |
| Prerequisite to future content – multiplication/ division | What knowledge or concepts do students need to multiply/divide fractions?
What helps students avoid misconceptions? |
Area pictures, like arrays from whole number multiplication, are the most meaningful. |
What Are the Fraction Models?
Let’s explore the set model, the length model, the number line model, and the area model.
The set model:
Students’ everyday experiences provide many opportunities to work with this visual image. For example, if they are asked to share these eggs between 2 people, they usually say that each group of 6 eggs is one-half of the total. However, it is difficult to link the symbol ½ to the situation until much later in school.

One of the most useful applications of the set representation involves fractions as operators (1/2 of 120 or ½ x 120). In this case, students will benefit from linking ½ x 120 to 120 ÷ 2. Also, finding the percentage of an amount of money involves the set model (25% of $200 is the same as ¼ of $200).
Use the set model where it is the most beneficial without leading to misconceptions.
| The most important part of a visual image for a fraction is the value of one whole. For the set model, the value of one whole is the total number of objects in the collection. |
The length model:
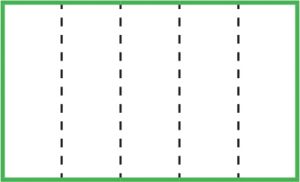
The first images of the length model usually involve a paper strip that is folded in one direction to show fractions (this example shows fifths). The advantage of this representation is the ability to extend it to add more units and therefore show fractions greater than one. The two strips below show four wholes (ones) and those same four wholes divided into fifths. This image is ideal for proper and improper fractions as well as linking to mixed numbers.
The length model is also perfect for addition and subtraction of fractions. Students can shade the strip to show the sum of 2 or more fractions and easily work with totals that are greater than one.
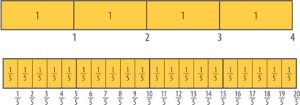
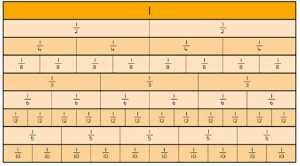
Addition and subtraction are straightforward when the denominators of the fractions are the same. The length model, extended to show several strips together, makes it easy for students to find equivalent fractions with denominators that are the same. This fraction wall shows that halves can be expressed as fourths, eights, twelfths or tenths; thirds can be written as sixths or twelfths; and so on. Additional walls can be attached to the right to show improper fractions. Extra rows can be included for other fractions.
| For the length model, the value of one whole is given by a defined length. |
The number line model:
The number line is closely related to the length model. With the number line, the focus becomes the distance from 0, but this can only occur when the distance from 0 to 1 is first established as being one whole. In this picture, the thirds can be determined after 1 has been marked on the number line.
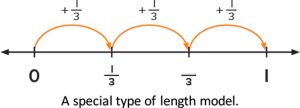
The number line provides the clearest picture linking fractions to whole numbers and shows the meaning of proper and improper fractions. Like the length model, the number line is good for establishing equivalence and for addition and subtraction of fractions. The number line is an abstract mathematical tool so students will, at first, lack everyday experiences with this valuable resource. It is always useful to work with the length model first and then transition students to the number line.
The number line helps with decimal fractions, too. In elementary grades, there are two main ideas that need to be established with rational numbers – rational number decimals represent fractions, and all decimals have a place value structure. The number line is ideal for showing both of these features.

| For the number line model, the value of one whole is given by the distance from 0 to 1. |
The area model:
The area model is, in some ways, similar to the length model. The folds and shading in the length model are one-directional on a rectangle shape. For the area model, almost any 2D shape can be used. The folds or shading are multi-directional. This representation may be more familiar to children than other models, but has more limitations.

It is difficult to clearly show improper fractions, which reduces the versatility of this model. Nevertheless, the area model can be used for equivalent fractions and addition/subtraction of fractions when the work is all less than one. Other models should be considered when making broader generalizations of fraction concepts.
| For the area model, the value of one whole is given by the total area of one 2D shape. |
Despite their utility, visual fraction models do not automatically guarantee success. Care needs to be taken with selecting an appropriate model based on the prior experiences of the students with fractions. It is also important to consider the fraction content that is being taught and use the model that will clearly display the desired ideas.
1 https://www.visiblelearningplus.com/content/research-john-hattie [Retrieved, 28 January, 2019]
2 https://study.com/academy/lesson/jerome-bruners-theory-of-development-discovery-learning-representation.html [Retrieved, 28 January, 2019]
3 Dienes’ name is often attached to the use of base-10 blocks, which are sometimes called Dienes’ blocks.




