Solving the Problem with Word Problems, Part III Multiplication & Division

Word problems are a principle way we teach mathematics in elementary school. From kindergarten through sixth grade students will encounter word problems that get progressively more difficult and complex. As I’ve travel around the country meeting with teachers, I often hear that word problems are a problem, for both teachers and students. So, I thought let’s do a four-part webinar series and accompanying blog posts on word problems. We explored some strategies for solving word problems, regardless of operation in our first post. In our second post, we looked specifically at the structure of addition and subtraction word problems. Today we’ll dive into the particulars of multiplication and division word problems.

Working Toward Mathematical Comprehension

But first a word about how we approach word problems in general. It’s essential that as students explore the world of word problems that they use one or more of five representations that include words, context, concrete objects, visual images, and symbols (read more about the five representations ). But more often than not, we ask students to go directly from reading the word problem to writing a symbolic equation, without ever giving them time and opportunity to explore the word problem from a context perspective or to make the math real by using manipulatives and images. This is what I often refer to spending time in the math sandbox (read more about the math sandbox). It’s here where students find the math in the problem and start to separate the math from the context, which helps them cement their mathematical comprehension. We can’t skip this stage, it’s here where students develop the thinking that helps them apply math in their everyday lives. And ultimately that’s the goal. The purpose of school mathematics isn’t to just learn basic math facts or do certain computations, but rather to prepare students to tackle the problems they encounter in the real world.

The Jobs of Multiplication & Division

I like to think about the operations as verbs, they act on things. They do specific work. They have specific jobs. And they do these jobs regardless of the type of number involved, be it whole numbers, fractions, decimals, or integers. I think this idea of the work of mathematical operations helps clarify the purpose of math for kids and helps them better understand what operation(s) to use when solving a particular problem. Multiplication and division have four basic jobs—equal groups, scaling, area/array, and combinations (counting principle).

Asymmetrical (Nonmatching) Factors

Equal Groups
Let’s look at the following problems.

• Problem A: The florist delivered 8 vases. There are 3 flowers in each vase. How many flowers are there?
• Problem B: The florist delivered 3 vases. There are 8 flowers in each vase. How many flowers are there?

In equal group situations, the two factors do different jobs. In the case above the first factor is the number of groups, vases in this case. The second factor is the size of each group, or number of flowers. The commutative property tells us that from a computation perspective that these two problems are the same, since both 8 x 3 = 24 and 3 x 8 = 24.

But I think these problems also demonstrate that multiplication is not always commutative from a meaning and context perspective. For example, let’s say that the above order is for a birthday party. The customer wants a vase as the centerpiece for each of 8 tables at the birthday party and has requested a total of 24 flowers. In this case, the florist needs to provide 8 vases with 3 flowers each so that there is a centerpiece for each of the 8 tables. Even though 3 vases with 8 flowers each also meets the customer’s order from a computation stand point, it doesn’t meet the customer’s expectation that there will be a centerpiece for each of the 8 tables. So, it’s critical that students not only do the math but that they understand that context can be important.

Using our same problems with vases and flowers, it’s important that students understand the notion of units, because this idea of equal groups in third grade becomes ratio and proportion in middle school. Let’s look at our same problem.

• 24 flowers = 8 vases x 3 flowers/vase

When you think about this in terms of units, that’s a proportion. If we add a ninth vase, how many more flowers do we need? 3 flowers. If we add a tenth vase, how many more flowers do we need? 3 flowers. We can see that this idea of equal groups feeds into ratio and proportion. It’s critical that students understand that multiplication is not just a shortcut for repeated addition, but that it’s really a different type of thinking and reasoning. Repeated addition is useful but proportional reasoning is more powerful as it addresses a wider range of situations.

The other power of unit, particularly for third graders who are learning about fractions, is that the denominator of a fraction is a unit of measure. So, when we take the time (and this is a substantial cognitive lift) to think about multiplication in terms of units, we are investing in students’ long-term mathematical growth, we are moving them towards proportional reasoning that they’ll explore in middle school. We don’t expect third graders to put these Ideas together, but it is important that we expose them to both proportional reasoning and multiplication in terms of units.

Scaling (Multiplicative Comparison)
Let’s look at the following problems.

• Problem A: Rajesh had 12 grapes for a snack. Latika had 3 more grapes in her snack that Rajesh did. How many grapes di Latika have?
• Problem B: Joyce lives 12 miles from her school. Andrew lives 3 times as far from the school as Joyce does. How far away from school does Andrew live?

If we think about the job of the “3” in each problem, its job is to increase in both problems, but it does it differently. In Problem A, it’s an Increase of 3 more, versus Problem B, where its 3 times as many. Kids will understand that idea of increasing before they understand the distinction between addition and multiplication. But we need to have this conversation with students that both situations are about an increase. Problem A is additive increase; Problem B is scaling (multiplicative) increase.

This concept of three times as far is a difficult one for students to grasp. It takes time for them and lots of rich math discourse for kids to truly understand scaling. Students need to spend time in the math sandbox working with concrete objects and visual images to truly understand the math involved. They need to have the opportunity to explain and defend their thinking and to listen to and critique their classmates’ ideas. Only then are students ready to turn the word problem into an equation.

In the scaling problem (Problem B) above, the factors are doing two different jobs. Joyce’s distance from school (12 miles) is the original value. Andrew’s factor, three times as far, is the scale or comparison relationship. The commutative property tells us that we can swap the numbers and get the same answer from a computation perspective. And this is important for students to understand. But, from a context perspective, Joyce living 12 miles from school and Andrew three times as far and Andrew living 3 miles from school and Joyce 12 times as far are different. In the first case, Joyce lives a lot further away from school than Andrew does in the second case. Again, it’s important that students understand that in the real world, swapping the values really changes the meaning.

Symmetrical (Matching) Factors

Area & Array
Let’s look at the following problem.

• Bradley bought a new rug for the hallway in his house. One side measured 3 feet, but he wasn’t sure what the other side measured because it was rolled up. The label said that it was a 36 ft2 rug. How long is the rug?

Up until now, we’ve been looking at multiplication with factors doing different jobs. But in the problem above, the factors do the same job, they are both measuring a linear dimension. However, here we are creating a new unit for the product. Feet times feet = square feet.

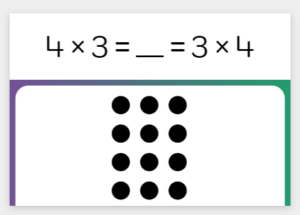
In real life, we use area & array to build spreadsheets, rows times columns = cells. Or we use it calculate area, as in the problem above. Or we use it calculate volume. For instance, In a rectangular prism, centimeters x centimeters x centimeters = cubic centimeters. And we need to make sure that students understand how these different calculations are connected. We typically use area or array Images In two ways while studying multiplication. In this example, we’re using area/arrays as problem structures. At other times, we might use an array image to show a computational principle for multiplication separate from any thought about problem structures.

Combinations (Counting Principle)
We’re going to look at the problem below, even though this type of problem typically isn’t introduced until middle school. However, it is another way we use multiplication. It’s another important job that multiplication performs.

• A deli offers 3 types of bread and 4 types of meat. How many different types of sandwiches can I make?

Here again we have different factors (bread and meat) and we are making a new product (sandwiches). In this case, 3 types of bread x 4 types of meat = 12 different sandwiches. Or you could have ice cream sundaes with a choice of 4 flavors, 2 sauces, and 3 toppings, so 4 x 2 x 3 = 24 different sundaes.

We’ve used multiplication to demonstrate the four different jobs that multiplication and division do, but any of the problems noted above can also be stated as a division problem. Additionally, we can vary the unknowns for each type of problem—equal groups, scaling, and area/array—that students encounter in elementary school. Typically, students work on problems where the product or resulting value is unknown. But it’s important that they also do problems where the unknown changes, as they will certainly encounter these problems on tests and of course in real life. You can actually use the same basic problem, but just move the unknown!